
N C = Force exerted on the ladder by the floor point Cĭraw a perpendicular from A on the floor BC. N B = Force exerted on the ladder by the floor point B The given question is illustrated in the figure below: The centre of gravity of the resulting flat body is R/6 from the original centre of the body and opposite to the centre of the cut portion. (The negative sign indicates that the centre of mass gets shifted toward the left of point O.) The relation between the centres of masses of two masses is given as, Let x be the distance through which the centre of mass of the remaining portion shifts from point O. (The negative sign indicates that this portion has been removed from the original disc.)

M (concentrated at O), and – M ′ concentrated at O′. Let O and O′ be the respective centres of the original disc and the disc cut off from the original.Īs per the definition of the centre of mass, the centre of mass of the original disc is supposed to be concentrated at O, while that of the smaller disc is supposed to be concentrated at O′.Īfter the smaller disc has been cut from the original, the remaining portion is considered to be a system of two masses.

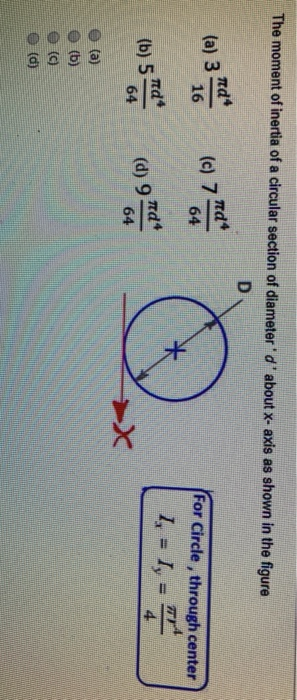
The word "MOI" stands for Moment of Inertia. Solve for the moment of inertia using the transfer formula.


 0 kommentar(er)
0 kommentar(er)
